Goal: define the limit of a function of two variables
The limit of a function of two variables is based on the limit of a function of one variable. Let's recall the definition:
Let f(x) be defined for all x≠a in an open interval containing a . Let L be a real number. Then
if for every ϵ> 0 there exists ẟ> 0 such that if 0< ❙x - a❙ < δ for all x in the domain of f, then
Definition
An open disk δ centered at a point (a, b) ε R² and of radius δ is defined by:
In a function of one variable, when x is is very close to a, we have ❘x-a❘ < δ meaning that the distance of all x to a is very small. In a disk, the distance of all circles are smaller than the square of the radius δIf we take the square of both sides and taking into account that the first side is strictly positive, we have:
Let f be a function of two variables x and y. The limit of the function f(x, y) as (x, y) approaches (a, b)) is L written as:
for every ϵ > 0, there exists ẟ > 0 such that
Limit rules
Let f(x,y) and g(x,y) be defined for all (x, y) ≠ (a, b) in a neighborhood around (a, b), and assume the neighborhood is completely inside the domain of f. Assume L and M are real numbers such that
Constant rule
Identity rules
Sum rule
Difference rule
Constant multiple rule
for all L if n is odd and positive and for all L⪈ 0 if n is even and positive for L≽ 0 for all (x,y) ≠ (a, b)
in neighborhood of (a, b)
Example
Find each of the following limits:
Let's first use the sum and difference rules to separate the terms:
Let's use the constant multiple rule on the second, third, fourth and fifth limit:
Let's use the identity rule on the first six limits and the the constant rule on the last limit:
b. In this example we need to make sure that the limit of the denominator is different of zero. Upon calculating the limit of the denominator we find:
Evaluate the following limit:

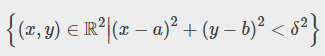














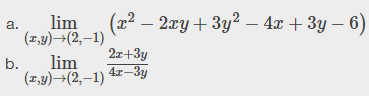







No comments:
Post a Comment