Objective:
Apply the method of integration by substitution to the resolution of definite integrals
Method (Recall)
The method by substitution consists in substituting the given variable by another variable
that makes the integral easier to calculate.
Let’s integrate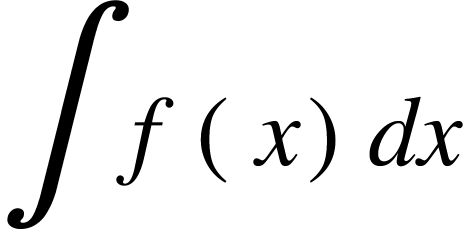 
by substitution.
by substitution.
If this integral can be written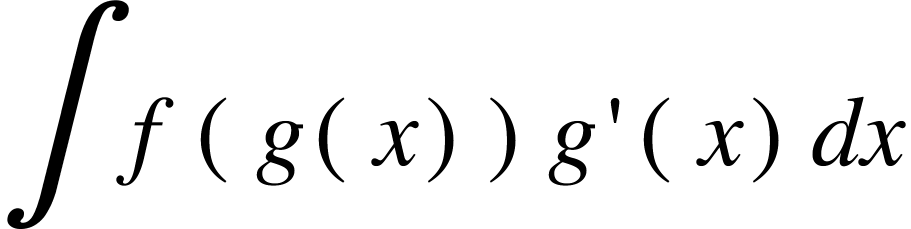 
with 
and 
then
with and then
The integral 
becomes easier to calculate. In the case of a definite integral
becomes easier to calculate. In the case of a definite integral
we have to calculate the new limits of integration for u.
Example I
Let’s calculate 
= 
= 
= 
= 
= 
=
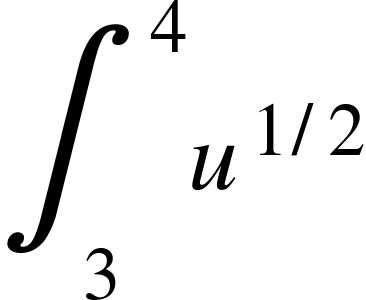
Example II Evaluate 
We can rewrite the expression as:
We have here 2 separate expressions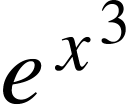
and x²
We seek to find out if the expression x² closed to dx is the derivative of the expression x³ in .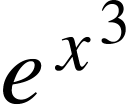
We notice that x² is almost the derivative of x³ . It misses the term 3. Therefore we have
to multiply and divide by 3 in order to have 3 x² . The given expression becomes:
We can rewrite the expression as:
We have here 2 separate expressions
and x²We seek to find out if the expression x² closed to dx is the derivative of the expression x³ in
. We notice that x² is almost the derivative of x³ . It misses the term 3. Therefore we have
to multiply and divide by 3 in order to have 3 x² . The given expression becomes:
represents a function composite of the exponential function f(x) = 
and the function g(x) = x³We can write
= f(g(x)). 3x represents the derivative of x³. We can write 3x².= . g'(x)Then we have:
If we do a change of variable by writing u = x³ du/dx = 3x² du = 3x²dx.= g'(x)dx
f(g(x)) =
. becomes f(u) = .
. There are new limits of integration for the new function of uIn the expresion u = x³, for x = 0 u = 0, for x = 2 u = (2)³ = 8
Let's substitute f(g(x)) by f(u) and g'(x)dx by du:
:
N.B. We have solved the second example by writing the given function as a composite function. We can skip these steps by doing the change of variable right away.as it was done in the first example,
Practice
