Civil engineers building roads in mountains use a topographical map that shows the different elevations of the mountain. Hikers walking through rugged trails use also a topographic map to show how steeply the trail changes. A topographical map contains curved lines called contour lines. Each contour line corresponds to the points of the map that have the same elevation. A level curve of a function of two variables f(x,y) is similar to a contour line in a topographic map.
Definition.
Given a function f(x,y) and a number c of its range, a level curve of the function with two variables f(x, y) for the value c is the set of points that satisfy the equation f(x, y) = c. A graph of the various level curves of a function with two variables is called a contour map.
Example
Let's consider a previous function:
This represents the equation of a circle of radius ⎷5 and centered at the origin. This is one level curve of the function. Choosing different values of c lead to other level curves. For c =3 and substituting this value in the function, we obtain x₂² + y² = 0, which is the origin. For c = 0, we have x₂² + y² =9, which is the circle centered at the origin and radius 3, For c = 1, we have x² + y² = 8, which is the circle centered at the origin and of radius 2⎷2. The contour map of the function is shown below:


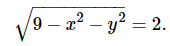



No comments:
Post a Comment